by Aleksander Mardinian (Guest Writer)
In mathematics, many of the elements performed are formulaic and repetitive. This is often what trips us up in exams, under pressure or when other things are on the mind. When unfocused or distracted, small errors can pop up resulting in more mistakes than we would normally make.

On occasions like this when the solution is hard to see, it is important to refocus and take a step back. Slowly revisiting the problem at hand, dismissing any assumptions we have made can help us get past a mental block. If the problem is perplexing us then we need to go back to the core principles and think about what we can and what patterns are emerging.
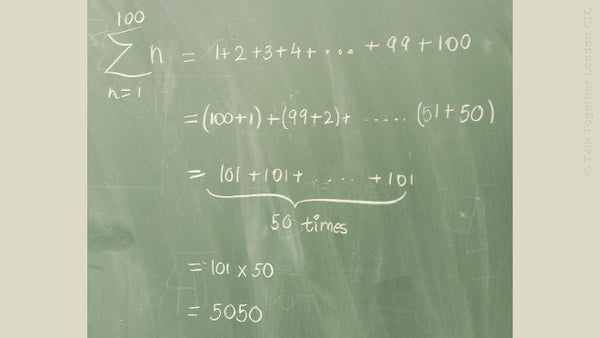
Some of the hardest problems in mathematics are solved using patterns whether this is the simplification of an infinite sum or finding the solution to a trigonometry problem. When the famous mathematician Carl Gauss was still at primary school, he was too smart and finished his assigned work too quickly. Therefore, his teacher told him to add all the numbers from 1 to 100 to keep him busy. After a few seconds, Gauss came back with the answer: 5050. He did this by finding a pattern: instead of adding the numbers sequentially (1+2+3+…) he added them up in pairs ([1+100]+[2+99]+…) this meant that the sum of 1 to 100 was actually just 50 times 101 = 5050. Obviously Gauss was very smart, but the principal of using patterns applies in all aspects of problem solving.

Other ways of dealing with mathematical errors are:
- Understanding where you most often trip up and paying particular attention to those processes
- Creating a fool-proof methodology for you to follow such as a list of tasks to follow
- Pulling out the important information from the problem statement, and focusing on what steps will get you from there to the required solution.
Each of these methods are related in that the problem simply needs to be broken down into smaller pieces and addressed individually before being put back together again. Not only will these methods reduce the number of errors that you make but the maths problems will also be easier to tackle!


